News of the discovery of unconventional superconductivity in a paramagnetic heavy-fermion system UTe₂ at the end of 2018 has excited strongly the researchers working on the condensed matter physics. Within a few years since the first report in arXiv, many new aspects have been unveiled both experimentally and theoretically. One of the intriguing phenomena in UTe₂ recognized in the very early stage is its large superconducting critical fields. For the magnetic fields applied along the hard magnetization b-axis, the superconducting transition can survive well above the Pauli paramagnetic limit [1,2]. This fact suggests the realization of the long-sought spin-triplet superconductivity. Such unconventional superconductivity is believed to realize in the ferromagnetic superconductors (FMSCs), such as URhGe and UCoGe. In the case of UTe₂, however, the ground state is paramagnetic. Here, we introduce our observation of metamagnetic transition in UTe₂ and discuss the relation between metamagnetism and superconductivity by compared to FMSCs [3].

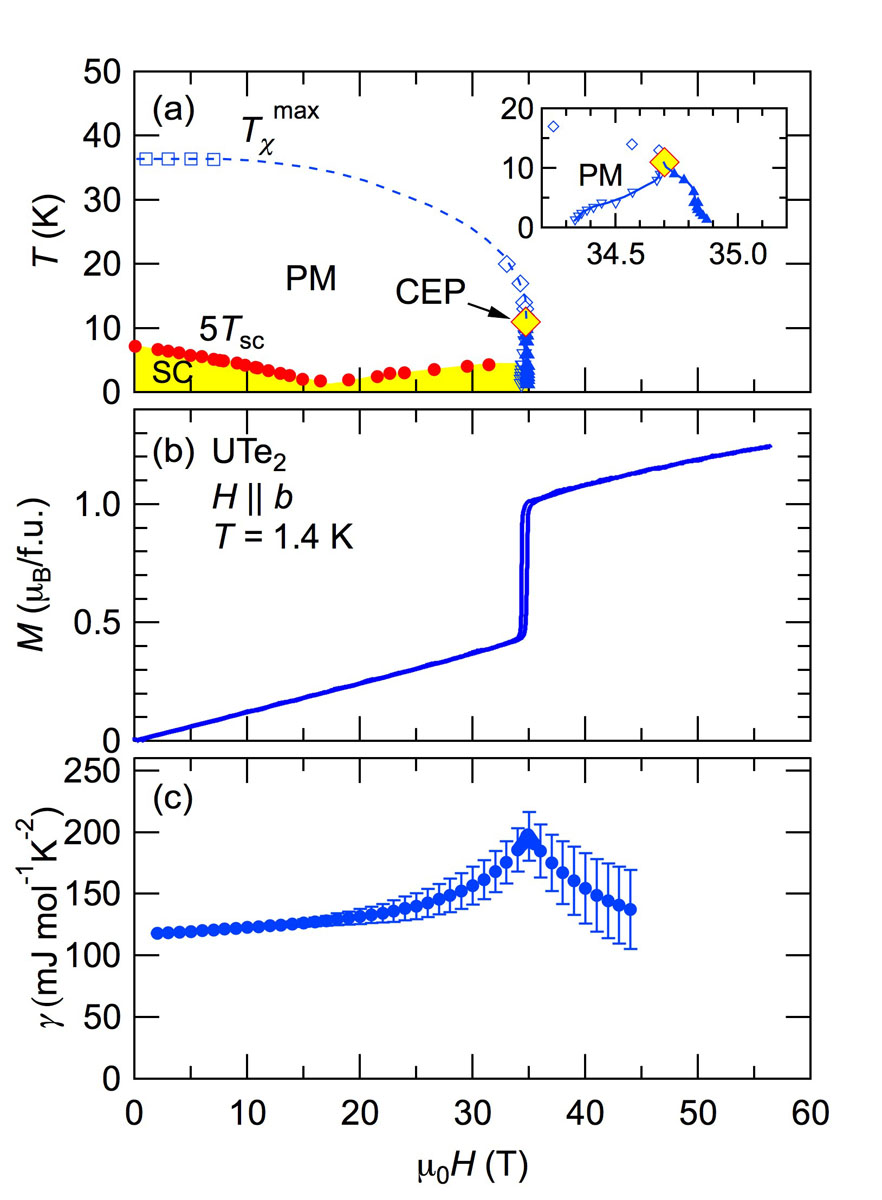
The high-field magnetization was measured by using the pulse magnets installed in the International MegaGauss Science Laboratory. Figure 1(b) shows the magnetization curve for the field along the b axis at 1.4 K. At μ₀H_m ~ 35 T, magnetization suddenly increases with a large step of ~0.6 μ_B. Because of the hysteric behavior against the magnetic field, this metamagnetic transition is of first-order. From the magnetization curves at various temperatures, we summarized a magnetic phase diagram of UTe₂ in Fig. 1(a). The field hysteresis disappears above 11 K, indicating the existence of the critical end point (CEP), and the transition changes to crossover. As known in many paramagnets, the energy scale of metamagnetic transition, μ₀H_m , corresponds to T_χ^max, where the temperature dependence of magnetic susceptibility shows a broad maximum. This relation is further supported by the fact that μ₀H_m (T) connects to T_χ^max(μ₀H). At almost the same time, the high-field magnetoresistance measurements independently revealed a similar phase diagram [4]. A sharp step-like increase in the resistance across the H_m may reflect the modification of the Fermi surface, although it is not clear yet. More interestingly, the superconducting (SC) phase shows a reentrant behavior above 16 T and is suddenly suppressed above μ₀H_m [5] [see Fig. 1(a)]. This unusual T_c enhancement can be explained qualitatively by a simple mass-enhancement model as follows. Figure 1(c) represents the magnetic-field evolution of the electronic specific heat g, derived by the thermodynamic Maxwell relation using the temperature dependencies of magnetization at constant fields [3]. With increasing fields, the γ increases and shows a maximum at H_m. This enhancement is also confirmed by the direct specific heat measurement using a pulse magnet in ISSP [6]. This enhancement on going to metamagnetic transition indicates that the development of the fluctuation evolves around H_m. This fluctuation may stabilize the SC phase even at high magnetic fields as has been adapted to explain the reentrant superconductivity in URhGe. Using a simplified McMillan-type formula, the field-enhancement of the superconducting transition temperature can be reproduced qualitatively [see Refs. 3 and 6 for more detail and references therein].

Fig. 1. (a) Magnetic phase diagram of UTe₂ for H // b determined by H_m and T_c^max. CEP denoted by a diamond is critical end point. (b) Magnetization curve for H // b at 1.4 K. (c) Magnetic field dependence of the electronic specific heat derived from magnetization curves by the Maxwell relation.

Now, it is no doubt that the high-field studies make an important role to reveal the superconductivity on UTe₂. Soon after our work [4], more exciting phenomena in high fields were reported [7]: Surprisingly, another SC phase appears at H_m when the field is applied intermediate angles between the a and c axis. This is in stark contrast to the fact of suppression of SC at H_m for the b axis. Our struggles continue.

References

• [1] S. Ran, C. Eckberg, Q.-P. Ding, Y. Furukawa, T. Metz, S. R. Saha, I.-L. Liu, M. Zic, H. Kim, J. Paglione, and N. P. Butch, Science 365, 684 (2019).
• [2] D. Aoki, A. Nakamura, F. Honda, D. X. Li, Y. Homma, Y. Shimizu, 
Y. J. Sato, G. Knebel, J.-P. Brison, A. Pourret, D. Braithwaite, G. Lapertot, Q. Niu, M. Vališka, H. Harima, and J. Flouquet, J. Phys. Soc. Jpn. 88, 043702 (2019).
• [3] A. Miyake, Y. Shimizu, Y. J. Sato, D. Li, A. Nakamura, Y. Homma, F. Honda, J. Flouquet, M. Tokunaga, and D. Aoki, J. Phys. Soc. Jpn. 88, 063706 (2019).
• [4] W. Knafo, M. Vališka, D. Braithwaite, G. Lapertot, G. Knebel, A. Pourret, J.-P. Brison, J. Flouquet, and D. Aoki, J. Phys. Soc. Jpn. 88, 063705 (2019).
• [5] G. Knebel, W. Knafo, A. Pourret, Q. Niu, M. Vališka, D. Braithwaite,
G. Lapertot, M. Nardone, A. Zitouni, S. Mishra, I. Sheikin, G. Seyfarth, J.-P. Brison, D. Aoki, and J. Flouquet, J. Phys. Soc. Jpn. 88, 063707
(2019).
• [6] S. Imajo, Y. Kohama, A. Miyake, C. Dong, J. Flouquet, K. Kindo, and D. Aoki, J. Phys. Soc. Jpn. 88, 083705 (2019).
• [7] S. Ran, I.-L. Liu, Y. S. Eo, D. J. Campbell, P. Neves, W. T. Fuhrman, 
S. R. Saha, C. Eckberg, H. Kim, J. Paglione, D. Graf, J. Singleton, and N. P. Butch, Nat. Phys. 15, 1250 (2019).

Authors

• A. Miyake, Y. Shimizu^a, Y. J. Sato^a, D. Li^a, A. Nakamura^a, Y. Homma^a, F. Honda^a, J. Flouquet^b, M. Tokunaga, and D. Aoki^{a, b}
• ^aTohoku University
• ^bCEA-Grenoble