Anomalous Nernst effect (ANE), a thermoelectric effect in magnetic material, has recently attracted attention as unique energy-harvesting technology. It holds promise in developing high-efficiency energy-harvesting devices with a simple lateral structure, high flexibility, and low production cost. The design of magnetic materials that exhibits a large ANE will help develop a novel energy harvesting technology. Here, we observed a high ANE exceeding 3 µV/K above room temperature in the kagome ferromagnet Fe₃Sn with a high Curie temperature of 760 K [1].

Figure 1a shows the temperature dependence of anomalous Nernst coefficient S_ij and transverse thermoelectric conductivity α_ji. With increasing temperature up to 400 K, the magnitude of the ANE coefficient increases monotonically and reaches 3 µV/K above room temperature. The temperature dependence of α_ij/T exhibits logarithmic behavior over almost a decade of temperature from 400 K down to 40 K, and remains roughly constant at ~0.02 Am^-1K^-2 below ~40 K (Fig. 1b). The logarithmic behavior of α_ij/T signals a nontrivial electronic structure close to the Fermi energy.

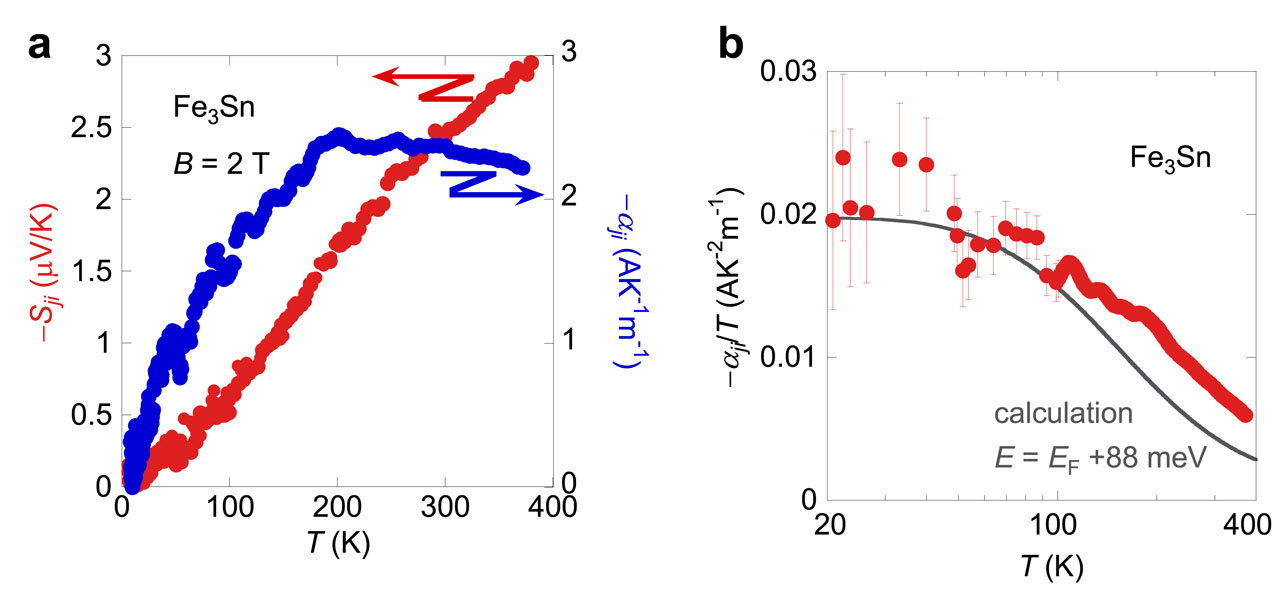
Fig. 1. (a) Temperature dependence of the Nernst signal (S_ji) and the transverse thermoelectric conductivity (α_ij) measured under an external magnetic field of 2 T. (b) Temperature dependence of the transverse thermoelectric conductivity (α_ji/T). The dark line represents α_ji/T obtained by DFT calculation at E_F = +88 meV.

To investigate the origin of ANE, we carried out first-principles calculations to analyze α_ij, and the calculated result agrees well with the experimental data (Fig. 1b). We then conclude that the origin of the ANE is a pair of partially flat bands of up- and down-spins, known as the “nodal plane” (Fig. 2a). Indeed, strong intensity of the Berry curvature arising from the flat nodal plane appears at specific chemical potentials (Fig. 2b). Similar to the nodal lines, nodal planes are ubiquitous in electronic band structures without SOC. Nodal planes often turn into nodal lines at constant energy cuts, and these constant energy lines lead to partially flat bands with a small energy gap after introducing SOC. Such characteristic band structure is not unique to Fe₃Sn and could be realized in other kagome lattice materials. It is well known that M points in kagome lattice may result in van Hove singularities (namely, the derivative of energy dispersion is zero). Therefore, energy dispersion on lines connecting M points could be flat compared with other k paths. Our theoretical analysis based on the first-principles calculation clarifies that a “nodal plane” induces strongly enhanced Berry curvature, resulting in the large ANE. The flat degenerate electronic bands provide a valuable guide to designing magnetic materials with a large ANE.

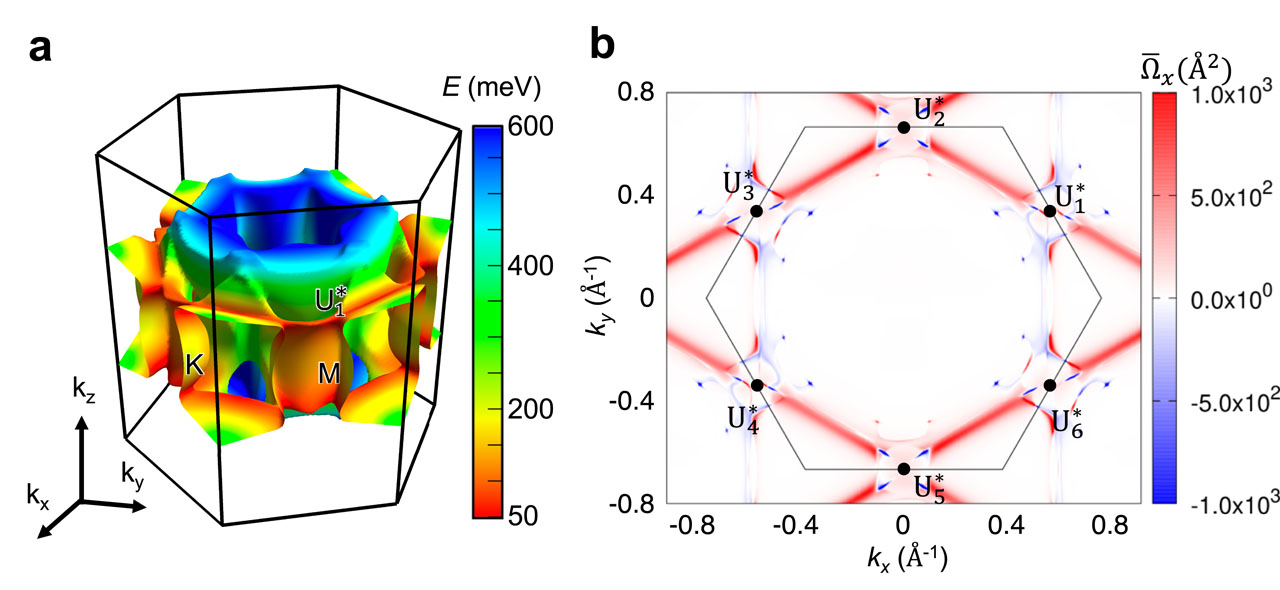
Fig. 2. (a) Nodal plane in the hexagonal Brillouin zone obtained as follows: Δε(k) ≡ ε^α(k) − ε^β(k) = 0, where Δε(k), ε^α(β)(k), and k are the energy difference between two bands, eigenvalue for up- and down-spin bands, and wave vector, respectively. The color bar shows the energy of the nodal plane calculated without SOC. (b) Contour plot of the sum of the Berry curvature over the occupied states (E = E_F + 50 meV) on k_z = 0.13 plane.

References

• [1] T. Chen, S. Minami, A. Sakai, Y. Wang, Z. Feng, T. Nomoto, M. Hirayama, R. Ishii, T. Koretsune, R. Arita, and S. Nakatsuji, Sci. Adv. 8, eabk1480 (2022).

Authors

• T. Chen, S. Minami^a, A. Sakai, Y. Wang^a, Z. Feng, T. Nomoto^a, M. Hirayama^b, R. Ishii, T. Koretsune^c, R. Arita^a,b, and S. Nakatsuji
• ^aThe University of Tokyo
• ^bRIKEN Center of Emergent Matter Science (CEMS)
• ^cTohoku University