Some magnetoelectric effects, including the Edelstein effect, often occur in the materials without some inversion symmetry. Materials exhibiting such cross-correlation are expected as various new functional materials. Recently, a new magnetoelectric effect is attracting attention in the metallic antiferromagnetic compound, in which the spatial inversion symmetry is broken at the magnetic site. This new cross-correlation is understood as a phenomenon that appears as a result of the realization of odd parity multipole [1, 2].

We discovered a new antiferromagnetic heavy fermion compound Ce₃TiBi₅ in which the local inversion symmetry at cerium site is broken (Figs. 1(a) and (b)). The crystal structure of Ce₃TiBi₅ is P6₃/mcm, and the global inversion symmetry is maintained. Therefore, the current-induced magnetization phenomenon in the sense of the Edelstein effect does not occur. Ce₃TiBi₅ has only one cerium site, and the cerium forms zigzag chains along the c-axis. The antiferromagnetic ordering temperature of T_N is 5.0 K, electrical resistivity, magnetic susceptibility, and specific heat show a distinct anomaly, respectively. Some odd parity multipoles are expected in the antiferromagnetic state, although the magnetic structure has not yet been determined (Fig. 1(c)).

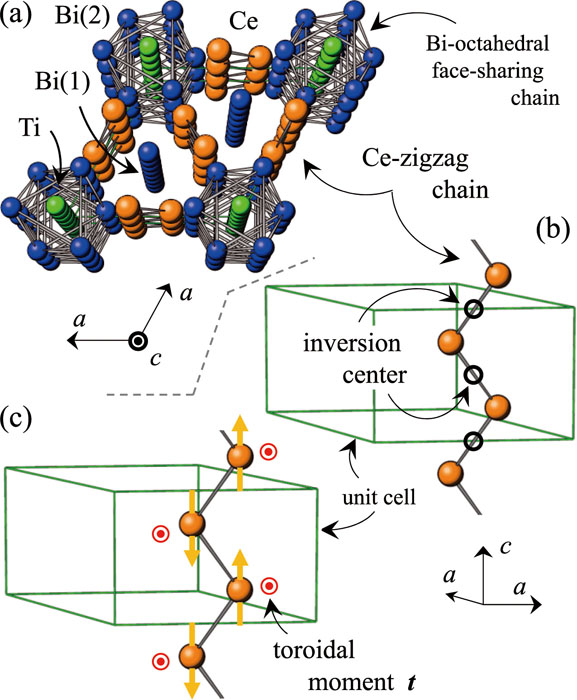
Fig. 1. (a) Top view of the crystal structure of Ce₃TiBi₅. (b) Schematic side view of the crystal structure, where only Ce ions on a front side are drawn for clarity. Green line denotes a unit cell. Black circles indicate the position of the inversion center. (c) Magnetic structure on the Ce-zigzag chain, where the unit cell does not change even at the ordered state. Orange arrows on the Ce ions indicate the expected ordered magnetic moments below T_N. The red mark denotes a toroidal moment on a Ce ion at the ordered state, which is a candidate as a realized odd multipole state on Ce₃TiBi₅.

We performed the magnetization measurements with applying several constant electric currents parallel to the c-axis of Ce₃TiBi₅ at around T_N using commercial magnetometer of MPMS. In this measurement, slight static magnetic field was applied to determine the sample position. Current-induced magnetization (M_ME) was obtained from subtracting magnetization induced by applying slight static magnetic field and zero electric current as blank magnetization.

We have succeeded in measuring the current-induced magnetization only on the antiferromagnetic state [3, 4]. Figure 2(a) shows the temperature (T) dependence of M_ME on Ce₃TiBi₅. It is found that M_ME(T) starts to appear just below T_N with decreasing T, although it is almost independent of temperature and is approximately zero above T_N. This T dependence was also observed at several electrical current (i), and then we show plots of M_ME versus i at 3.0 K in Fig. 2(b) to discuss the i dependence of the magnitude of M_ME. M_ME shows the linear i dependence, which suggests that M_ME on Ce₃TiBi₅ linearly increases with an increase in the electric field.

Fig. 2. (a) Comparison of the T dependence of the M_ME between several i for sample A. (b) i dependences of M_ME at 3 K for samples A and B. (c) Comparison of the T dependence of M_ME between different H. (d) H dependence of M_ME at 3 K.

Next, T dependence of M_ME at several magnetic field (H) is shown in Fig. 2(c). M_ME(T) exhibits an identical curve despite a difference in the magnitude of H. The magnitude of M_ME at each magnetic field and T = 3.0 K was decided from the M_ME(T) at several magnetic fields (5 ≤ H ≤ 40 Oe) and is plotted in Fig. 2(d). The magnitude of M_ME is independent of H in this field range, which indicates that M_ME has a similar value even in zero magnetic field.

The current-induced magnetization was observed only below the antiferromagnetic transition temperature of 5.0 K. This current-induced magnetization exhibits linear dependence with respect to the DC electric current and demonstrates little dependence on the magnetic field, i.e., the behavior persists even in zero magnetic field. Based on these results, we suggest that the current-induced magnetization originated from the magnetoelectric effect of the odd multipole state on the Ce-zigzag chain structure. Meanwhile, the sign and magnitude of the slope of i dependence of MME has large sample dependence as shown in Fig. 2(b). We consider the sample dependence to be explained by the imbalance of the domain structure of the AFM state. However, we do not know what kind of domain structure is realized in the antiferromagnetic state. We are conducting experiments with an interest in the consistency between the realized odd parity multipole state on Ce₃TiBi₅ and the current-induced magnetization measurements with various geometry.

References

• [1] S. Hayami, H. Kusunose, and Y. Motome, Phys. Rev. B 90, 024432 (2014).
• [2] H. Saito, K. Uenishi, N. Miura, C. Tabata, H. Hidaka, T. Yanagisawa, and H. Amitsuka, J. Phys. Soc. Jpn. 87, 033702 (2018).
• [3] M. Shinozaki, G. Motoyama, M. Tsubouchi, M. Sezaki, J. Gouchi, S. Nishigori, T. Mutou, A. Yamaguchi, K. Fujiwara, K. Miyoshi, and Y. Uwatoko, J. Phys. Soc. Jpn. 89, 033703 (2020).
• [4] H. Saito, H. Amitsuka, G. Motoyama, and M. Shinozaki, Solid State Physics 55, 611 (2020).

Authors

• M. Shinozaki^a, G. Motoyama^a, J. Gouchi, S. Nishigori^a, T. Mutou^a, A. Yamaguchi^b, K. Fujiwara^a, K. Miyoshi^a, and Y. Uwatoko
• ^aShimane University
• ^bUniversity of Hyogo