Steps as a Disorder on Atomic-Layer Superconductors
Hasegawa Group
Two-dimensional (2D) superconductors, whose thickness is thinner than the coherence length, exhibit peculiar properties. Compared with bulk superconductors, 2D superconductors are susceptible to disorder since induced electron localization enhances repulsive electron-electron interaction, which suppresses the Cooper pairing. As demonstrated with thickness-dependent critical temperature of amorphous superconducting thin films, changing parameters such as disorder, thickness, and perpendicular magnetic field lead to a superconductor-insulator transition (SIT). The zero-temperature transition is one of the typical examples of quantum phase transitions, and has been investigated extensively to elucidate its universal properties.
Recent technical advancements have allowed us to fabricate highly crystalline 2D superconductors, some of which has only mono-atomic layer thickness. In the case of such highly-ordered 2D superconductors it has been reported that during the SIT induced by the magnetic field application metallic phase appears in between. So far, the properties of this peculiar quantum metallic phase have been investigated mainly by transport measurements but the mechanism is still controversial. In order to understand its details atomistic real-space measurements are crucial. In the present study, we studied superconducting properties of striped-incommensurate (SIC) phase of the Pb/Si(111) system; one of the monoatomic-layer superconductors, formed on vicinal Si substrates. Since the substrate is tilted, uniformly distributed steps, whose spacing is equivalent or even smaller than the coherence length, should behave as a dispersed disorder on the ultimately thin superconductor. We investigated how the steps affect the superconducting properties and the magnetically induced SIT by using low-temperature scanning tunneling microscopy (STM).
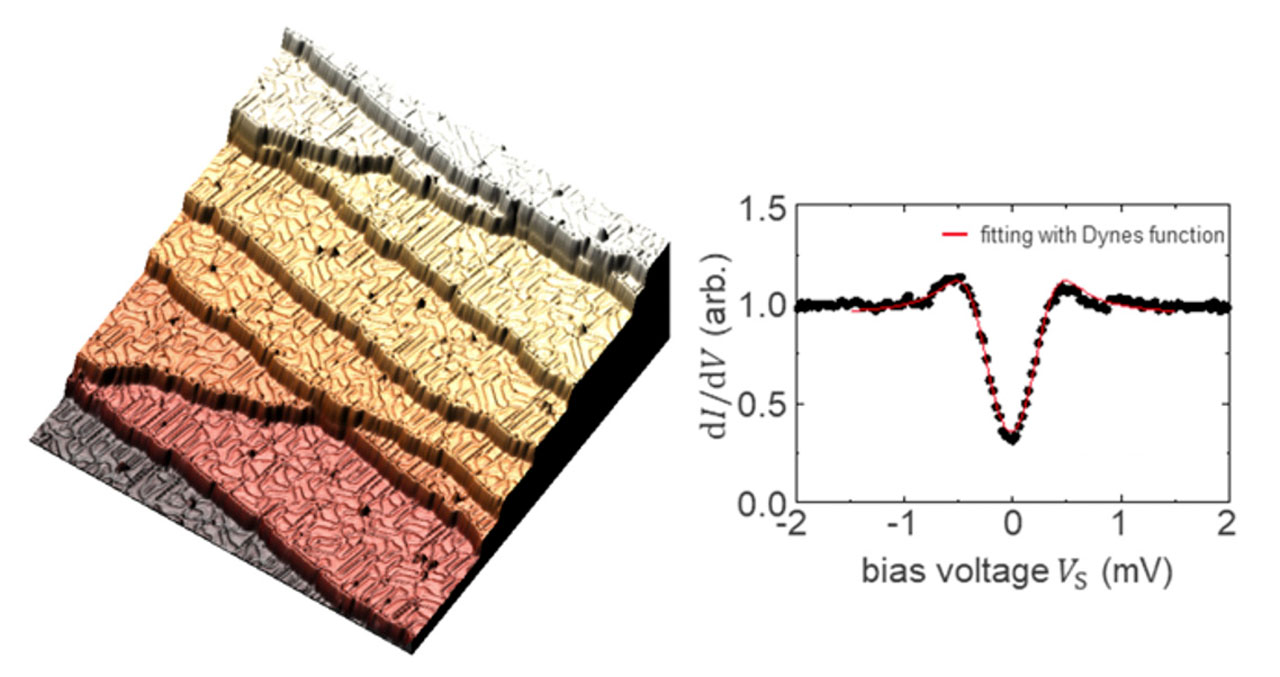
Figure 1 shows a 3D-rendered STM image of the SIC phase formed on a 1.1°-tilted vicinal Si(111) substrate taken at 0.36 K. A characteristic SIC stripe pattern can be seem on the terraces. In the image, the vertical scale is severely exaggerated; the step height corresponds to 0.31 nm whereas the averaged terrace width is 16 nm. A tunneling spectrum taken on a terrace shows a superconducting gap that can be well fitted with the Dynes function. The fitted superconducting gap, 0.30 meV, is almost same as that taken on SIC phase formed on a flat substrate, indicating the Cooper pairing is not affected by the presence of the steps.
In our previous studies on the role of steps on superconductivity, we found that the steps basically behave as a Josephson junction connecting the neighboring terraces, and the strength of the decoupling strongly depends on the phases [1, 2]. Among the Pb monolayer phases, the steps of the √3×√43 phase decouple terraces significantly to make the terraces superconducting nano stripes [2]. On the other hand, the decoupling of the SIC phase is rather weak so that vortices are just pinned at step edges. In the case of the vicinal one, as the terrace width (16 nm) is much shorter than the coherence length of the flat one (38 nm), more than one steps are involved on the formation of a vortex.
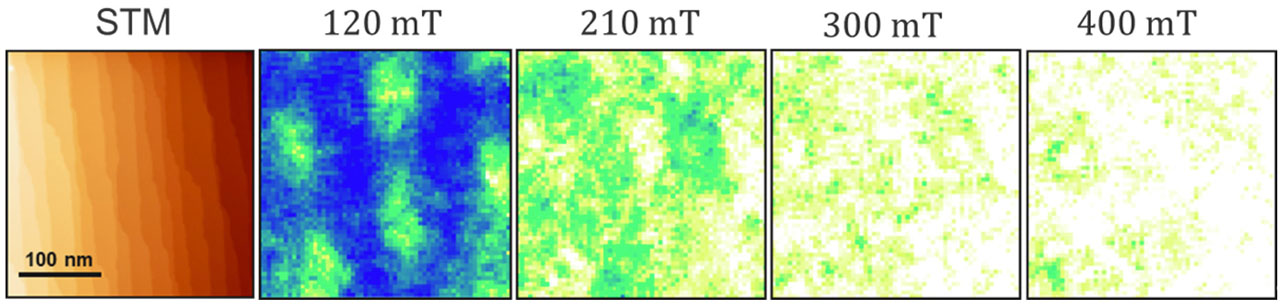
Figure 2 shows an STM image and zero-bias-voltage maps, which correspond to the spatial distribution of the density of states (DOS) at the Fermi level, taken under various magnetic fields. At 120 mT, the vortices are observed elongated along the step direction. The DOS at the core of the vortices is not fully recovered to that of the normal metal. These are the characteristic features of an Abrikosov-Josephson vortex. The oval shape of the vortices is due to the reduced coherence length along the step-crossing direction caused by the limited mean free path due to the presence of the steps. Because of the reduced coherence length, the critical magnetic field, which is inversely proportional to the coherence length, is enhanced on the vicinal superconductor.
At the magnetic field of 210 mT, the number of vortices increases as well as the background DOS. The vortices are no longer distinct, and significant corrugation in DOS is discernible. By further increasing the magnetic field, DOS gradually increases and its distribution becomes homogeneous. Around 330 mT, DOS exhibits saturation, indicating the breaking of superconductivity. According to the transport measurement performed on the same phase the vortex liquid phase and quantum metallic phase are observed at these magnetic fields. The observed blur vortices and inhomogeneous DOS contrast presumably corresponds to thermally activated or quantum diffusing vortex liquid phase.
References
- [1] S. Yoshizawa et al., Phys. Rev. Lett. 113, 247004 (2014).
- [2] F. Oguro et al., Phys. Rev B 103, 085416 (2021).