The shape of the Metal Phthalocyanine TPP[M(Pc)(CN)₂]₂ compound (M = 3d metal) is unique; two CN groups are perpendicularly attached to the center of the M(Pc) planar molecule. In these compounds, the magnetic d electron of the central ion in the planar molecule and the conducting π electron reside in the same unit molecule. This fact makes the interaction between d and π electrons important. In M = Fe, the compound exhibits a giant negative magnetoresistance (GMR); when a high magnetic field is applied perpendicularly to the c-axis, the resistivity drops by more than two orders of magnitude at low temperatures. On the other hand, M = Mn, GMR is hardly seen. The motivation of our research is to know the difference in magnetic structure between compounds that GMR can be seen and compounds that GMR cannot be seen.

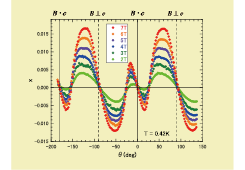
Fig. 1. Torque curves under various magnetic fields when the magnetic field was rotated in a plane included the c-axis at 0.42 K.

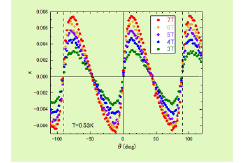
Fig. 2. Torque curves under various magnetic fields when the magnetic field was rotated in the ab plane at 0.53 K.

Here we report the results of our torque magnetometry experiments on M = Mn compound, TPP[Mn(Pc)(CN)₂]₂, using the microcantilever for the atomic force microscopy (AFM). This technique is very powerful, because even if the sample mass is on the order of 1 μg, it is so sensitive that we can detect the torque signal. In our experiments only tiny sample is available. The sample was mounted at the tip of the cantilever and the magnetic field orientation dependence of the signal that is nonlinearly proportional to the torque was measured.

In Figs. 1 and 2, typical torque curves measured when the magnetic field was rotated in the plane that includes the c-axis, and when the magnetic field was rotated in the ab plane, are depicted, respectively. A twofold symmetric signature with large and small peaks is seen in Fig. 1, which has never been observed in our former measurements on TPP[Fe(Pc)L₂]₂ (L = CN, Br, and Cl) family. This is characteristic of the ferromagnetism. On the other hand, a sawtooth waveform and fourfold-symmetric behavior can be seen in Fig. 2. This feature is interpreted as the antiferromagnetism. The electrons responsible for these behavior should be d electrons of the Mn³+ ion for the former case, and π electrons for the latter case. At first sight, it would be thought of that the electron we observe by our torque measurements might be the magnetic d electron only, in whichever plane the magnetic field was rotated. However, our former studies have already clarified that although the anisotropic one-dimensional Heisenberg (AODH) model which should describe only the behavior of the d electron can reproduce the feature of the torque signal with the magnetic field rotated in the plane including the c-axis, it is insufficient to describe the behavior of the torque when the magnetic field is rotated in the ab plane, in terms of the AODH model only. This model should be supplemented by the antiferromagnetic model of π electrons, because otherwise the behavior of torque signals in this field rotation plane cannot be reproduced at the region of low temperatures and low magnetic fields. We are thus allowed to consider that the electron we can observe when the field rotated in the ab plane should be the π electron, that is different from the electron when the field rotated in the plane including the c-axis. We are thus able to separate contributions due to the d and π electrons.

Now we also have to take the susceptibility data into account. That shows the weak antiferromagnetic behavior of the d electron as a result of applying the Curie-Weiss law. We have to reconcile the torque and the susceptibility data. One possibility is that the d electron should be in the canted antiferromagnetic state, instead of the ferromagnetic. Even in the canted antiferromagnetic case, the torque curve is also the same as that in the ferromagnetic case if the anisotropy energy is very small. We also pointed out that the experimental observation that the magnetoresistance is hardly seen is consistent with the canted antiferromagnetic model. The canted antiferromagnetism is very likely, because the canted antiferromagnetism might be mediated by the Dzyaloshinsky-Moriya interaction. In fact, the space inversion symmetry is broken and the spin-orbit coupling plays an important role in our sample.

References

• [1] K. Torizuka, Y. Uwatoko, M. Matsuda, G. Yoshida, M. Kimata, and H. Tajima, J. Phys. Soc. Jpn. 86, 114709 (2017).

Authors

• K. Torizuka^a, M.Matsuda^b, and Y. Uwatoko
• ^aNippon Institute of Technology
• ^bKumamoto University