Tuning the Spin Hall Effect of Pt from the Moderately Dirty to the Superclean Regime
Otani Group
Spintronics research relies on the techniques of spin currents generation and detection. Therefore the discovery of the spin Hall effect was one of the most important breakthrough in this area, which enabled us to interconvert between charge and spin currents without using ferromagnets. Thanks to the discovery, spin Seebeck effect [1] and spin pumping [2] have been demonstrated successfully.
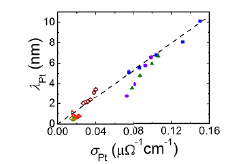
Fig. 1. Spin diffusion length λPt of Pt depending on the longitudinal conductivity σPt. Each symbol shows a different sample which is measured in various temperatures. The black dashed line is the linear fitting. This linearity is evidencing that the spin relaxation mechanism in Pt is governed by Elliot-Yafet mechanism. The obtained variation of the spin diffusion length λPt covers the values in the previous researches.

Fig. 2. Spin Hall angle θSH,Pt of Pt depending on the longitudinal conductivity σPt. The inset shows the Spin Hall conductivity σSH,Pt of Pt depending on the longitudinal conductivity σPt. Each symbol shows a different sample which is measured in various temperatures. The black solid line is a guide to the eye which express the inverse proportional relation between θSH,Pt and σPt when you consider only intrinsic mechanism. The purple dashed line is the corrected line from the black solid one with considering extrinsic contribution. On the left part (Moderately dirty region) the behavior of the θSH,Pt is well explained only by intrinsic contribution. On the other hand on the right part (Superclean region) θSH,Pt gradually differ from the black line and extrinsic contribution become more dominant.
The SHE was theoretically predicted by Dyakonov and Perel in 1971 [3] and revisited by Hirsch in 1999 [4]. It is widely recognized that the SHE in nonmagnetic materials shares the same mechanism with the anomalous Hall effect (AHE) in ferromagnets; either intrinsic or extrinsic mechanism. In case of the AHE, experiments with changing the longitudinal conductivity of ferromagnetic metals have been performed, showing that the intrinsic and extrinsic contributions scale differently with the longitudinal conductivity. In contrast to the AHE, however, a systematic experimental study on the SHE has been still lacking.
We have studied on the spin diffusion length λPt and the spin Hall angle θSH,Pt (conversion yield between spin and charge currents) of platinum in a wide range of conductivities σPt by means of the spin absorption method using lateral spin valve devices [5]. Pt was chosen because it is a prototypical SHE metal so that our results can be compared with many reported values. Also there have been a discussion on the vales of λPt and θSH,Pt because the value differ in each report where λPt ranges from 1~10 nm and θSH,Pt from 1~10 % [6]. A linear relation between λPt and σPt was firstly observed as shown in Fig. 1, evidencing that the spin relaxation in Pt is governed by the Elliott-Yafet (EY) mechanism. Also, we decomposed intrinsic and extrinsic contributions for each sample by analyzing the data measured in different temperatures. We found a single intrinsic spin Hall conductivity (σSHint = 1540 ± 100 Ω-1cm-1), that is the material’s characteristic value, for Pt in all the studied range of σPt, being in good agreement with the theoretical value [7]. By collecting all the data in our study, we have obtained for the first time the crossover from the intrinsic regime (moderately dirty) to the extrinsic one (superclean) in the SHE by changing the quality of Pt as shown in Fig. 2, equivalent to that for the AHE. Our results explain the wide dispersion in previously reported values of λPt and θSH,Pt, and show a route to maximize the spin Hall angle. The smaller is σPt (dirtier Pt), the larger will be θSH,Pt.
References
- [1] K. Uchida, J. Xiao, H. Adachi, J. Ohe, S. Takahashi, J. Ieda, T. Ota, Y. Kajiwara, H. Umezawa, H. Kawai, G. E. W. Bauer, S. Maekawa, and E. Saitoh, Nat. Mater. 9, 894 (2010).
- [2] E. Saitoh, M. Ueda, H. Mikajima, and G. Tatara, Appl. Phys. Lett. 88, 182509 (2006).
- [3] M. I. Dyakonov and V. I. Perel, Phys. Lett. A 35, 459 (1971).
- [4] J. E. Hirsch, Phys. Rev. Lett. 83, 1834 (1999).
- [5] E. Sagasta, Y. Omori, M. Isasa, M. Gradhand, L. E. Hueso, Y. Niimi, Y. Otani, and F. Casanova, Phys. Rev. B 94, 060412(R) (2016).
- [6] J. Sinova, S. O. Valenzuela, J. Wunderlich, C. H. Back, and T. Jungwirth, Rev. Mod. Phys. 87, 1213 (2015).
- [7] T. Tanaka, H. Kontani, M. Naito, T. Naito, D. S. Hirashima, K. Yamada, and J. Inoue, Phys. Rev. B 77, 165117 (2008).
