In ferromagnetic (FM) conductors, an electric current may induce a transverse voltage drop in zero applied magnetic field: this anomalous Hall effect is observed to be proportional to magnetization M, and thus is not usually seen in antiferromagnets in zero field. Recent developments in theory and experiment have provided a framework for understanding the anomalous Hall effect using Berry-phase concepts [1], and this perspective has led to predictions that, under certain conditions, a large anomalous Hall effect may appear in spin liquids and antiferromagnets without net spin magnetization [2]. Although such a spontaneous Hall effect has now been observed in a spin liquid state [3], a zero-field anomalous Hall effect has hitherto not been reported for antiferromagnets.

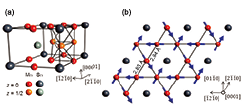
Fig. 1. (a) The crystallographic unit cell of Mn₃Sn. (b) An individual ab-plane of Mn₃Sn. Mn moments form an inverse triangular spin structure. Each Mn moment has the local easy-axis parallel to the in-plane direction towards its nearest-neighbor Sn sites.

Fig. 2. (a) Field dependence of the Hall resistivity ρ_H and the longitudinal resistivity ρ at 300 K in the magnetic field B || [2-1-10] with the electric current I || [01-10]. (b) Field dependence of the non-collinear AF ordering driven Hall resistivity ρ_H^AF = ρ_H − R₀B − Rsµ₀M at 300 K.

Mn₃Sn is a hexagonal antiferromagnet (AFM) that exhibits non-collinear ordering of Mn magnetic moments at the Néel temperature of T_N ~ 420 K [4]. The system has a Ni₃Sn-type structure with space group P6₃/mmc (Fig. 1(a)). The basal plane projection of the each ab-plane consists of a slightly distorted kagome lattice of Mn, and the associated geometrical frustration manifests itself as an inverse triangular spin structure that carries a very small net FM moment of ~ 2 mµ_B/Mn (Fig. 1(b)) [4]. All Mn moments lie in the ab-plane and form a chiral spin texture with an opposite vector chirality to the usual 120° structure. This spin configuration has an orthorhombic symmetry, and only one of the three moments in each Mn triangle is parallel to the local easy-axis. Thus, the canting of the other two spins towards the local easy-axis is considered to be the origin of the weak FM moment [4].

We have carried out detailed transport measurements using high-quality single crystals of Mn₃Sn to reveal the properties associated with the non-collinear antiferromagnetic (AF) ordering [5]. Figure 2(a) presents the field B dependence of the Hall resistivity, ρ_H(B) at 300 K for B || [2-1-10]. ρ_H(B) exhibits a clear hysteresis loop with a sizable jump of |Δρ_H| ~ 6 μΩcm. This is strikingly large for an AFM, and is larger than those found in elemental transition metal ferromagnets (FMs) such as Fe, Co and Ni [1, 6]. Notably, the sign change occurs at a small field of ~ 300 Oe. In contrast, the longitudinal resistivity ρ(B) remains constant except for spikes at the critical fields where the Hall resistivity jumps (Fig. 2(a)). Correspondingly, the Hall conductivity, σ_H = − ρ_H/ρ², for in-plane fields along both [2-1-10] and [01-10] shows a large jump and narrow hysteresis. For instance, with B || [01-10], σ_H has large values near zero field, ~ 20 Ω⁻¹cm⁻¹ at 300 K and nearly 100 Ω⁻¹cm⁻¹ at 100 K. This is again quite large for an AFM and comparable to those values found in FM metals [1, 7]. On the other hand, the Hall signal for B || [0001] shows no hysteresis but only a linear field dependence.

If we label the additional term originating from the non-collinear AF ordering as ρ_H^AF, the Hall resistivity in Mn₃Sn can be described by ρ_H = R₀B + Rsµ₀M + ρ_H^AF.By subtracting R₀B and Rsµ₀M from ρ_H, we find that ρ_H^AF is nearly independent of B or M, unlike what is found in FMs, as shown in Fig. 2(b). With the reversal of a small applied in-plane field, ρ_H^AF changes sign, corresponding to the rotation of the staggered moments of the non-collinear spin structure [4]. Thus, the large AHE, ρ_H^AF, must have a distinct AF-driven origin. This soft response of the large anomalous Hall effect in an AFM could be useful for various applications including spintronics—for example, to develop a memory device that produces almost no perturbing stray fields.

Finally, we note that the present exceptionally large AHE found in an AFM with vanishingly small magnetization indicates that a large fictitious field due to Berry phase must exist in momentum space, and is expected to generate various effects including orbital ferromagnetism and the spin Hall effect. Exploration of such effects and their external-field control are suitable subjects for future studies.

References

• [1] N. Nagaosa et al., Rev. Mod. Phys. 82, 1539 (2010).
• [2] R. Shindou and N. Nagaosa, Phys. Rev. Lett. 87, 116801 (2001); H. Chen et al., Phys. Rev. Lett. 112, 017205 (2014); J. Kübler and C. Felser, Europhys. Lett. 108, 67001 (2014).
• [3] Y. Machida, S. Nakatsuji, S. Onoda, T. Tayama, and T. Sakakibara, Nature 463, 210 (2010).
• [4] S. Tomiyoshi et al., J. Phys. Soc. Jpn. 51, 2478 (1982); P. J. Brown et al., J. Phys. Condens. Matter 2, 9409 (1990).
• [5] S. Nakatsuji, N. Kiyohara, and T. Higo, Nature 527, 212 (2015).
• [6] C. L. Chien and C. R. Westgate, The Hall Effect and its Applications (Plenum, 1980).
• [7] T. Miyasato et al., Phys. Rev. Lett. 99, 086602 (2007).

Authors

• S. Nakatsuji, N. Kiyohara, and T. Higo